Solution)
class Solution:
def getMinimumDifference(self, root: Optional[TreeNode]) -> int:
# Initialization
prev, res = None, float("inf")
# Depth-First-Search
def dfs(node):
# Base Case
if not node:
return
# Adjusting the scope
nonlocal prev, res
# Left Subtree
dfs(node.left)
# Recursive Call
if prev:
res = min(res, node.val - prev.val)
prev = node
# Right Subtree
dfs(node.right)
dfs(root)
return res
Problem: LeetCode
Reference: NeetCode
Time Complexity: O(n)
Space Complexity: O(1)
*n = number of nodes
Explanation)
As we're given a bst (binary search tree), we'll use an inorder traversal to compare nodes in a sorted order.
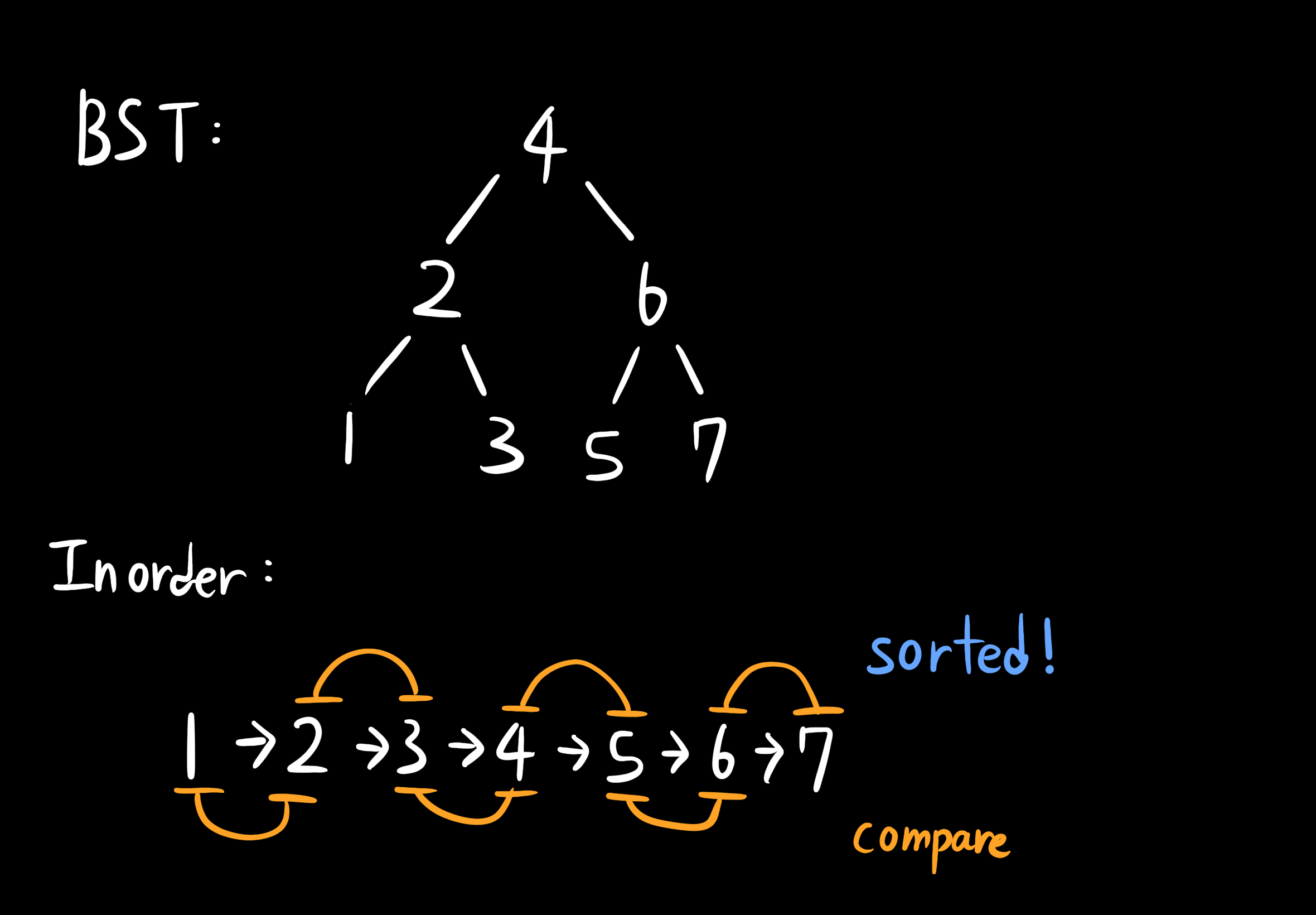
With the inorder traversal, we can find the minimum difference by comparing the difference between the adjacent nodes.
Initialization)
To compare the adjacent nodes while traversing the tree, you need a variable that saves a previous node.
Also, have a variable that will save the minimum differences while traversing. The initial value will be a positive infinity because when we compare the first difference with the initial res, we want to make sure that the first difference will replace the positive infinity.
def getMinimumDifference(self, root: Optional[TreeNode]) -> int:
prev, res = None, float("inf")
Base Case)
If there isn't a node in a tree, a difference doesn't exit thus we'll simply return.
def getMinimumDifference(self, root: Optional[TreeNode]) -> int:
prev, res = None, float("inf")
def dfs(node):
if not node:
return
Nonlocal)
Since our dfs() function is an inner function and we're trying to use variables that are defined between functions, we have to adjust the socpe of the variables (especially when we're trying to adjust the values).
So let's use the keyword nonlocal to use prev and res in an inner function.
def getMinimumDifference(self, root: Optional[TreeNode]) -> int:
prev, res = None, float("inf")
def dfs(node):
if not node:
return
nonlocal prev, res
Recursive Calls)
Since we're doing an inorder traversal, the order of the recursive calls would be
1) left subtree
2) root node
3) right subtree
When the root node step is reached during the recursion, it'll check if prev is not Null (since the initial value was Null) then find and compare the differences.
Once the smaller difference is decided, we'll update prev to the current root node, because we need to find the difference between the current root node and the future adjacent node.
Call this dfs() function and return res.
def getMinimumDifference(self, root: Optional[TreeNode]) -> int:
prev, res = None, float("inf")
def dfs(node):
if not node:
return
nonlocal prev, res
dfs(node.left)
if prev:
res = min(res, node.val - prev.val)
prev = node
dfs(node.right)
dfs(root)
return res
TMI)
With this problem, I learned how you have to switch the steps in recursion according to which traversal you're using. I'm still confused with when I have to use a helper function. Hopefully solving these problems will eventually help me master binary tree / recursion :]
'[LeetCode] > [Easy]' 카테고리의 다른 글
| [Top Interview 150] 67. Add Binary (0) | 2023.07.20 |
|---|---|
| [Top Interview 150] 35. Search Insert Position (0) | 2023.07.19 |
| [Top Interview 150] 637. Average of Levels in Binary Tree (0) | 2023.07.17 |
| [Top Interview 150] 226. Invert Binary Tree (0) | 2023.07.13 |
| [Top Interview 150] 100. Same Tree (0) | 2023.07.11 |
